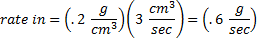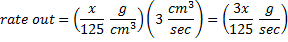Differential Equation, Drug mixture in blood stream

Blood goes into an organ at a rate of 3 cm^3/sec (or cm3/sec) and leaves at the same rate. The organ has a liquid volume of 125 cm^3 (or cm3). A drug is introduced into the blood going into the organ at a concentration of 0.2 g/cm^3. Find a formula for the concentration of the drug at time t (t is time in sec after it is first introduced). When will the concentration of the drug in the organ reach 0.1 g/cm^3?

We will let x = grams of the drug, t = tine in seconds and the rate is g/sec

Follow the steps below to solve.

This is a differential equation mixture problem. We can set our initial equation based on (rate in) - (rate out) = dx/dt (or change of x over change in time)
We know our rate in as (.2 g/cm^3)(3 cm^3/sec). This does give us our correct rate units of g/sec):
Our rate out is (x/125 g/cm^3)(3 cm^3/sec). x/125 is the amount of drug in the organ at time t. This is always changing and therefore we assign it a variable:
Now simplified with only our values:
Collecting like terms to get the dx and the x together and then setting up the integral:
Integrate both sides. The left side is integrated as follows:
The completed integration equation is shown and the C (constant) has been collected on one side:
We can use the known initial condition x(0)=0, to solve for C. We know x(0)=0 because at time 0 there was no drug in the organ.
Rewriting the equation with our constant, C:
Because of the natural log, ln, we will raise each side to the power of e and we are also multiplying each side by (-3) to be able to solve:
Simplifying with using the laws of logarithms and exponents: Also note, we can drop the absolute value sign as the constraint of our problem requires it to be positive.
This only gives us x at time t, or the amount of the drug in grams. The question asks for the concentration of the drug at time t. To find concentration we divide this number by 125 (total capacity) to find the concentration:
Therefore, our concentration of the drug at time t is:
Find t when the concentration of the drug reached 0.1 g/cm^3 and simplifying:
Taking the natural log of both sides and solving for t:
Final answer for t is approx 28.88 seconds:

Find a function f such that f'(x) = x^3 and the line x + y = 0 is tangent to the graph of f.

The problem states:

Find a function f such that f'(x) = x^3 and the line x + y = 0 is tangent to the graph of f.

Follow steps below to solve (C is the unknown constant):

Use x + y = 0 and solve for y we get y=-x:
Take the derivative of y and we see that y' = -1.
Since f'(x) = y', we can use y' = -1 to solve for x by saying -1 = x^3 and solving for x we get x = -1:
Using x = -1 in x + y = 0 we can find that y = 1:
Take the antiderivative  of f'(x) and we get f(x) = (1/4)x^4 + C
Because we know x and y, we can use those to solve for C:
Using C and the antiderivative gives us our final answer for our function f:

Solve the Differential Equation: y'=(3x^2)/(y^3) where y(1)=2

Solve the differential equation y'=3x^2/y^3 where y(1)=2:

Rewrite with dy/dx:
Multiply both sides by y^3
Multiply both sides by dx. We now have our variables separated and ready to integrate:
Integrating both sides:
Using y(1)=2 to solve for C, we find that C = 3.
Our new equation with C:
Multiplying both side by 4:
Taking the 4th root of both sides to solve for y gives us our final answer:

Integrate the following integral: x sin(x)

Find the following indefinite integral:

This integral is easily solved by using the Integration by Parts formula. Follow the steps below to solve:

Reciting the Integration by Parts equation:
Set u=x and dv=sin(x) dx and solve for du and v:
Plugging in u, du, v and dv into the IBP equation above:
Simplify and then integrate the remaining integral we arrive at our final answer:

The final answer is -xcos(c)+sin(x)+C: